
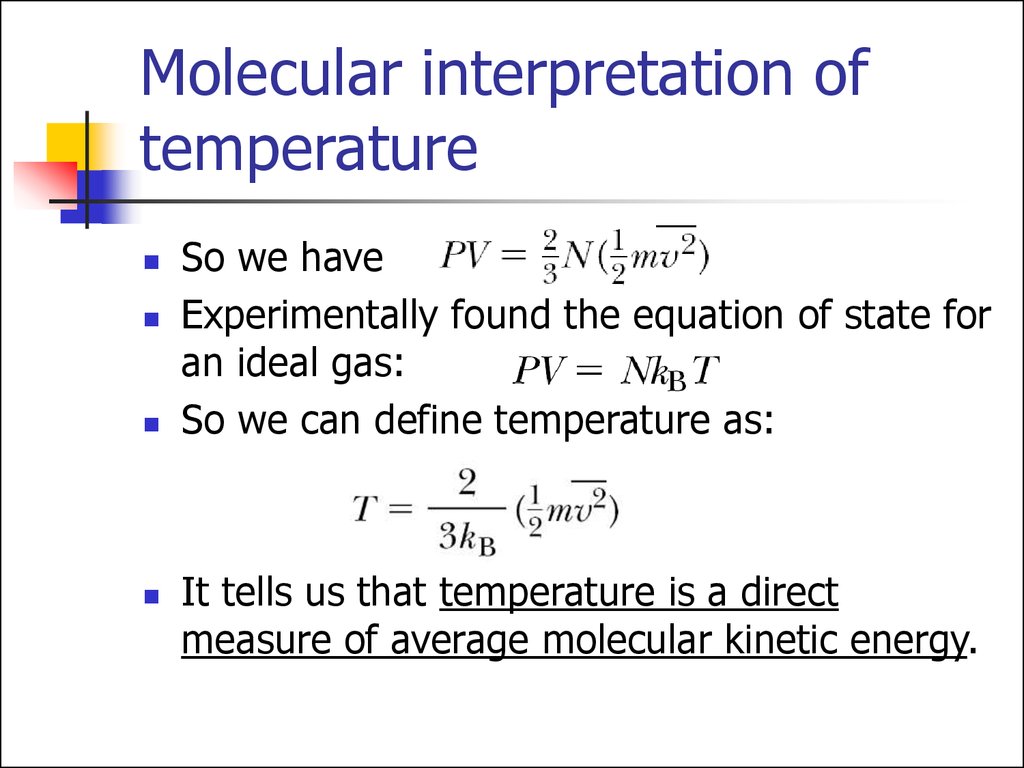
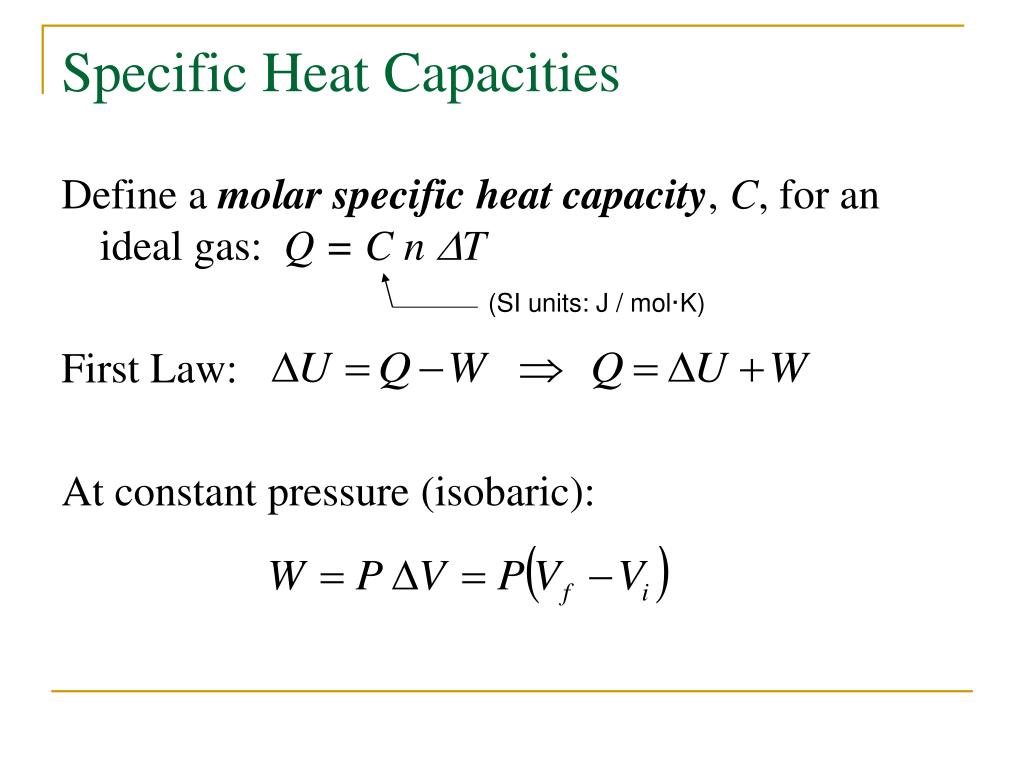
Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983). See also: Mayer’s formula Isochoric process – main characteristics The pressure is directly proportional to the Kelvin temperature for a fixed mass of gas at constant volume. See also: Specific Heat at Constant Volume and Constant Pressure. Since there is no work done by or on the system, the first law of thermodynamics dictates ∆U = ∆Q. Where the property c v (J/mol K) is referred to as specific heat (or heat capacity) at a constant volume because under certain special conditions (constant volume), it relates the temperature change of a system to the amount of energy added by heat transfer.
According to the ideal gas model, the internal energy can be calculated by: Since the process is isochoric, dV = 0, the pressure-volume work is equal to zero. Pressure-volume work by the closed system is defined as:
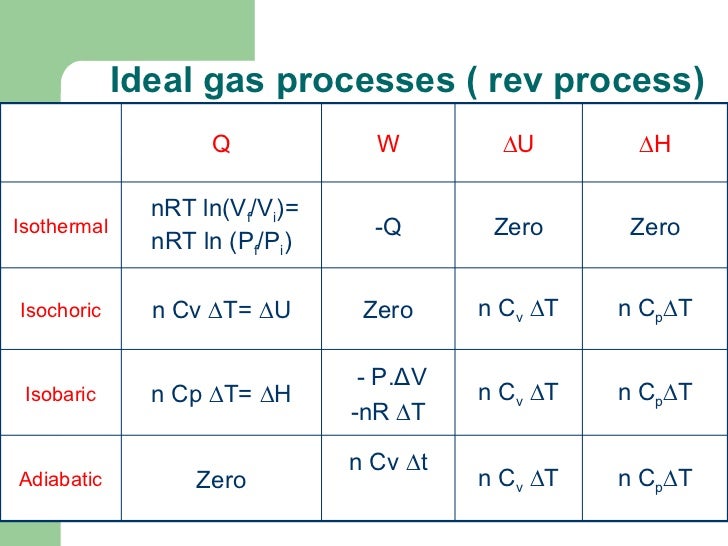
On a p-V diagram, the process occurs along a horizontal line with the equation V = constant. The isochoric process can be expressed with the ideal gas law as: In this equation, the symbol R is the universal gas constant that has the same value for all gases-namely, R = 8.31 J/mol K.
Let assume an isochoric heat addition in an ideal gas. The case n ➝ ∞ corresponds to an isochoric (constant-volume) process for an ideal gas and a polytropic process. Since the volume remains constant, the heat transfer into or out of the system does not the p∆V work but only changes the system’s internal energy (the temperature). It describes the behavior of gas inside the container that cannot be deformed. An isochoric process is a thermodynamic process in which the volume of the closed system remains constant (V = const).


 0 kommentar(er)
0 kommentar(er)
